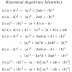Mathematics Formula Algebra - Identities

What is a formula? We can say 'Any conventional or fixed methods based on experiences for doing something' or literally 'a set form of words, as for stating or declaring something definitely or authoritatively, for indicating procedure to be followed, or for prescribed use on some ceremonial occasion' is the general meaning of the formula. Mathematically we can express formula as 'A principle or rule which is frequently expressed in the language of Mathematically symbols'.

Similar to other physical science, computational, and engineering subjects mathematics is also has varied and the vast list of formulas. Classification of formulas can be done according to standard (Class) or Branches of Mathematics. In my opinion, it is beneficial to classify on the basis of  Branches of Mathematics is more useful so we can classify such as

1. Mathematics Formula Algebra 
2. Mathematics Formula Geometry
3. Mathematics Formula Commercial mathematics
4. Mathematics Formula Mensuration
5. Mathematics Formula Trigonometry
6. Mathematics Formula Coordinate Geometry
7. Mathematics Formula Statistics & Probability
8. Mathematics Formula Calculus 
9. Mathematics Formula Miscellaneous 

Mathematics Formula Algebra 

In algebra, we have various topics having formulas and identities, Some are listed below:- 

• Algebraic Identity
• Exponents and Powers
• Least Common Multiple (LCM) & Highest Common Factor(HCF)

Now we shall write about algebraic identities.

Algebraic identities are algebraic equations that are true for all the variables involving in it. Algebraic identities are also an integral part of  Formula in Algebra. These identities contain various constants and variables on both sides of the equation. When we factorize a polynomial, we often use these algebraic identities. On the basis of terms of identities, it can be divided into two parts.

• Binomial Algebraic Identity
• Trinomial(Polynomial) Algebraic Identity

Binomial Algebraic Identity

We know that the algebraic expression having two terms is known as Binomial. The few basic important algebraic identities frequently used in algebra as well as other branches of mathematics are such as( Instead of a and b we can use any alphabets of many languages). 

Trinomial(Polynomial) Algebraic Identity

The algebraic expression having three terms is known as Trinomial. The few basic important algebraic identities frequently used in algebra as well as other branches of mathematics are such as( Instead of a and b we can use any alphabets of many languages). 

The First Four Binomial Algebraic Identities are in the syllabus of Middle Standard (Class 6 to 8) While all remaining along with Trinomial(Polynomial) Algebraic Identityare used in secondary Standards in mathematics.

We can use the above identities to solve problems, Few illustrations are such as

          Similarly, we can use the above-mentioned identities to factorize algebraic polynomials and evaluate values like examples 3 and 4.

From the above illustration 8, we can conclude that identity second is obtained by first with substitution of b by (-b). Similar results can be obtained for five and six, seven & eight.

Note:- The number of identities is not fixed they are written only for convenience.

Aims for mathematics education at the elementary stage & Secondary stage

Aims or the main goal for mathematics education in schools is the mathematisation of the child’s thinking using various strategies/techniques. 
if we consider a real situation that is similar to most of us (Maybe up to 99.99 % in my opinion) that, Among all the school subjects, maximum emphasis is given by any chid to learning mathematics. We must all have experienced, during our school days and also being a teacher(Now), the extent of pressure exerted on children to perform at a higher level in mathematics in comparison with other subjects like Languages, Sciences, Social Sciences, Music & art, etc. Although the parents, irrespective of their educational status (including the first-generation Learner), suppress their children very hard to perform well in mathematics and perhaps maximum study time at home is spent on doing homework and creative activity (Learning of Tables is common phenomena for Parents up to 5 /10 /15 or 30 for brilliant students) in mathematics than in any other subject. 
Aims or the main goal for mathematics education in schools  may differ according to the opinion of persons but rigorously may be similar like 

At Elementary Stage

• At the most basic level, mathematics involves counting objects, recognizing numbers, and performing simple operations like addition, subtraction, multiplication, and division. 
• In the primary grades, students should be master these skills including general concepts of maths like factors, multiples, and classification of numbers and we have to provide numerous opportunities instead of rote learning methods. 
• Upon exiting at elementary stage (primary school), children should be comfortable with writing and identifying numbers, rote counting forward and backward & comparing numbers and quantities. 
• They should also be able to add, subtract, multiply, and divide numbers, and knowing about vice versa of basic operations.
• Clarity of thought and pursuing assumptions to logical conclusions is the main aim of learning mathematics.
• There are various ways of thinking, and the kind of thinking one learns in mathematics is an ability to handle to approach about  problem-solving.
•  The school mathematics must be activity-oriented for the learner.

At Secondary Stage

• To develop the powers of logical thinking and reasoning in the learner. 
• To Shifting the focus of mathematics education from achieving ‘narrow’ goals to ‘higher’ goals to the learner.
• To solve mathematical problems of daily life by mathematisation of the learner. 
• To understand and acquainted with the environment and culture with mathematisation.
•  To prepare the learner for various technical and auspicious future professions.
•  To prepare the learner for higher study. 
• To develop in the learner the power for invention 

The general aim of Mathematics Education

• To engage every student with a sense of success, while at the same time offering conceptual challenges to the emerging mathematician.
• To Changing modes of assessment to examine students mathematisation abilities rather than procedural knowledge continued from ancient times.
•  Enriching teachers with a variety of mathematical resources from time to time. 

As per position paper on 'Teaching of mathematics' by national focus group(NCF ) In India by NCERT are such as

• The mathematisation of the child’s thought processes.
•  Curriculum may be ambitious, coherent, and teaches important mathematics.
• School mathematics must be activity-oriented.
• Children learn to enjoy mathematics.
• Children learn important mathematics.
• Children see the importance of mathematics in various ways as something to talk about, to communicate, to discuss among themselves, to work together on.
• Children pose and solve meaningful problems.
• Children understand the basic structure of mathematics.
• Teachers expect to engage every child in the class.

Therefor to know about the aim of mathematics Education is very important before learning and inculcating among children or Mathematics lovers fraternity.

Mathematics Symbols are very important to learn mathematics, on using symbols we can easily understand. 

 Mathematics Symbols can be categorized on their importance in the following ways:

1. Mathematics symbols - Number
2. Mathematics symbols - Basic
3. Mathematics symbols - Algebraic
4. Mathematics symbols - Geometric
5. Mathematics symbols - Trigonometry
6. Mathematics symbols - Set Theory
7. Mathematics symbols - Statistics & Probability
8. Mathematics symbols - Calculus And Analysis
9. Mathematics symbols - Logical
10. Mathematics symbols - Roman
11. Mathematics symbols - Greek
12. Mathematics symbols - Miscellaneous

Now we shall learn /discuss Fourth topic Mathematics symbols - Geometric on this post.

Mathematics symbols - Geometric

 These are very important Basic Tools frequently used by us in algebra as well as in other branches of mathematics and sciences.

Symbol	NAME OF SYMBOL	DEFINITION / MEANING	ILLUSTRATION
∠	Angle	An angle is formed by two rays	∠ABC = 60°
	Measured angle		ABC = 30°
	Zero Angle	An angle of measure zero degree	∠ABC = 0°
∟	Right angle	An angle of measure ninety degrees = 90°	α = 90°
°	Degree	1 turn or round = 360°	α = 60°
deg	Degree	1 turn or round  = 360 deg	α = 60 deg
′	Prime ( Read as different from prime numbers)	Arcminute, 1° = 60′	α = 60°59′
″	Double prime	Arcsecond, 1′ = 60″	α = 60°59′59″
	Line	Infinite line	Drawn from any point
AB	Line segment	The lines from point A to point B	The lines from point B to point A is written as BA
	Ray	The lines that start from any point like A
	Arc	an arc from point A to point B	Part of the circumference of a circle of any measure = 60°
⊥	Perpendicular	Those lines intersect at right angles are perpendicular lines (90° angle).	AC ⊥ BC
∥	Parallel	Those lines do not intersect each other are parallel lines.	AB ∥ CD
≅	Congruent to	Those geometric figures having same shapes and sizes.	∆ABC≅ ∆XYZ
~	Similarity	Those geometric figures having the same shapes but sizes may be different.	∆ABC~ ∆XYZ
Δ	Triangle	Triangle shape	ΔABC≅ ΔBCD
|x-y|	Distance	Distance between  any two points x and y	| x-y | = 2 or 3 or any number
π	Pi constant	π = 3.141592654... is the ratio between the circumference and diameter of a circle and it is an irrational numbers	c = π⋅d = 2⋅π⋅r where r is the radius of circle and d = 2r
rad	Radians	Radians angle unit	360° = 2π rad or 2π c
c	Radians	Radians angle unit	360° = 2π c   or  2π rad
grad	Gradians	Grads angle unit	360° = 400 grad or g
g	Gradians	Grads angle unit	360° = 400 g or grad

Note:- Degree, Gradians and Radians are units of measurements of angles in various systems.
On having knowledge of the above Mathematics symbols - Geometric we can easily enhance our subject ideas.
________________________________________________

Mathematics symbols - Algebraic

Mathematics Symbols are very important to learn mathematics, on using symbols we can easily understand. 

 Mathematics Symbols can be categorized on their importance in the following ways:

1. Mathematics symbols - Number
2. Mathematics symbols - Basic
3. Mathematics symbols - Algebraic
4. Mathematics symbols - Geometric
5. Mathematics symbols - Trigonometry
6. Mathematics symbols - Set Theory
7. Mathematics symbols - Statistics & Probability
8. Mathematics symbols - Calculus And Analysis
9. Mathematics symbols - Logical
10. Mathematics symbols - Roman
11. Mathematics symbols - Greek
12. Mathematics symbols - Miscellaneous

Now we shall learn /discuss Third topic Mathematics symbols - Algebraic on this post.

Mathematics symbols - Algebraic

 These are very important Basic Tools frequently used by us in algebra as well as in other branches of mathematics and sciences.

Symbol	Name of Symbol	Definition / Meaning	Illustration
Any alphabets like x or y or z	Variable x or variable y or etc...	The unknown value we have to find	If 4x = 4, then x = 1 or  If -12z = 48, then z = -4
≡	Equivalence (Used in Relation)	Identical to	a ≡ b, a is identical to b.
≜	Equal by definition	Equal by definition (that is given)	This symbol is also used for the same:=
:=	Equal by definition	Equal by definition (that is given)	This symbol is also used for the same ≜
~	Approximately equal	Weak approximation	11.0001 ~ 10.9999
≈	Approximately equal	Approximation (more near in comparison to ~ )	tan x≈ x if x is very small tan(0.001) ≈ 0.001 or  sin x≈ x if x is very small
∝	Proportional to	Proportional to ( If number varies in definite proportion)	y ∝ x when y = k x, where k is a proportionality constant.
∞	Lemniscate	Symbol of Infinity	it can be also used in both sense + ∞ and - ∞.
≪	Much less than	Much less than (Very)	1 ≪ 1000000
≫	Much greater than	Much greater than (Very)	1000000 ≫ 1
( )	parentheses	calculate expression inside first	-2 × (3+5) = -16
[ ]	brackets	calculate expression inside first	[(1+3)×(1+5)] = 24
{ }	Braces	Used for a set or known as Curley Bracket also	A = {a, e, i, o, u}
⌊x⌋	floor brackets	Rounds number to lower integer	⌊5.1⌋ = 5 =⌊5.9⌋  = ⌊5.7⌋ =⌊5.3⌋
⌈x⌉	Ceiling brackets	rounds number to upper integer	⌈4.3⌉ = 5
n!	Factorial / Exclamation mark	In mathematics used for Factorial Notation	1! =1 2! = 1*2= 2 3! = 1*2*3 = 6 4! = 1*2*3*4 = 24 ... n! = 1*2*3*4 ...   continued products upto n
| x |	Vertical bars	Absolute value in Real numbers and Modulas in Complex Numbers	| -4 | = 4 or | -3.2 | = 3.2 or | -101 | = 101.
f (x) or g(x)	function of x	Mappings values of x to f(x)	f (y) = 4y-3
(f ∘ g)	Composition of functions	(f ∘ g) (x) = f (g(x))	f (x)=3x,g(x)=x-1 ⇒(f ∘ g)(x)=3(x-1)
(a,b)	Interval Open	(a,b) = {y | a < y < b} Initial and final values are not incliuded	y ∈ (0,6)
[a,b]	Interval Closed	[a,b] = {y| a ≤ y ≤ b} Initial and final values are also incliuded	y ∈ [-4,4]
∆	Delta	Difference ( Used in Numerical Analysis and higher studies)	∆t = t1 - t0
∆	Discriminant	Δ = b2 - 4ac in quadratic equation where   a = coefficient of square of x b = coefficient of x  c = constant term	Used for finding Nature of Roots - Quadratic Equations
∑	Sigma used for Summation	Used for summation - the sum of all values in the range of series	∑ xi= x1+x2+...+xn
∑∑	sigma	double summation
∏	capital pi	Used for Product - Product of all values in range of series	∏ xi=x1∙x2∙...∙xn
e	Euler's number	e = 2.718281828...	e = lim (1+1/y)y , Where y→∞
	e constant	For logarithms	e = lim (1+1/y)y , Where y→∞